3.4: Common Fractions Review

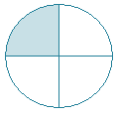
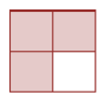
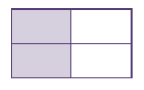
  1. Write fractions from the pictures.
    1. Circle cut in four equal parts. Three parts are blank. One segment is shaded to fill.
    2. Circle divided in three equal parts. Two parts are shaded to fill. One segment is blank.
    3. Square divided in four equal parts .Three parts are shaded to fill. One segment is blank.
    4. Rectangle divided in two parts. One segment is shaded to fill. One segment is blank.
    5. Rectangle divided in four equal parts. Two parts are shaded and two are blank.
    6. Long rectangle divided in 10 segements. Two segements are blank. Eight parts are shaded to fill.

     

  2. Draw your own fractions.
    1. [latex]\dfrac{2}{5}[/latex]
    2. [latex]\dfrac{3}{4}[/latex]
    3. [latex]\dfrac{4}{9}[/latex]
    4. [latex]\dfrac{1}{6}[/latex]
    5. [latex]\dfrac{1}{3}[/latex]
    6. [latex]\dfrac{7}{10}[/latex]

     

  3. Write the following fractions in words.
    1. [latex]\dfrac{1}{2}[/latex]
    2. [latex]\dfrac{1}{4}[/latex]
    3. [latex]\dfrac{3}{4}[/latex]
    4. [latex]\dfrac{21}{25}[/latex]
    5. [latex]\dfrac{1}{3}[/latex]
    6. [latex]\dfrac{5}{6}[/latex]

     

  4. Answer the questions using a common fraction.
    1. Suzie jogged 20 minutes out of 1 hour. What fraction of the hour did she jog? (Remember, 1 hour = 60 minutes.)
    2. Oliver planted 30 garlic cloves in September. 25 shoots have come up in the spring. What fractions of garlic bulbs did not grow a shoot?
    3. The class usually had 8 students, but 6 did not come on Monday. What fraction of students did not come?
    4. Stephen made 60 Easter cookies. His brother ate 3, and his mom ate 3 more. What fraction of cookies were eaten by Stephen’s family?
    5. Save On Foods grocery store sold 300 dozen eggs in one week. They had 450 dozen in stock. What fraction of the stock was sold?
    6. The test was out of 32. Sasha got 30 marks. What was her score?

     

  5. Compare the following fractions.
    1. [latex]\dfrac{3}{4}[/latex]      [latex]\dfrac{1}{4}[/latex]
    2. [latex]\dfrac{9}{10}[/latex]      [latex]\dfrac{3}{10}[/latex]
    3. [latex]\dfrac{1}{5}[/latex]      [latex]\dfrac{3}{5}[/latex]
    4. [latex]\dfrac{3}{8}[/latex]      [latex]\dfrac{7}{8}[/latex]
    5. seven tenths        three tenths
    6. one quarter        three quarters
    7. four fifths       three fifths
    8. one twelfth        eleven twelfths

     

  6. Identify each fraction by writing: proper fraction, improper fraction, or mixed number to each fraction.
    1. [latex]\dfrac{1}{2}[/latex]
    2. [latex]\dfrac{100}{47}[/latex]
    3. [latex]\dfrac{5}{6}[/latex]
    4. [latex]\dfrac{51}{2}[/latex]
    5. [latex]42\dfrac{1}{4}[/latex]

     

  7. Fill in the missing parts of the chart (see Table 3.4.1).
    Table 3.4.1: Question 7 Fill in the Table
    Improper
    Fraction    		 Mixed
    Number    		 Drawing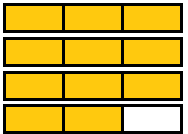
    — — 4 rectangles each divided into 3 parts. 3 rectangles are fully shaded. One rectangle has 2 parts shaded.
    [latex]\dfrac{29}{6}[/latex] — —
    — [latex]2\dfrac{3}{4}[/latex] —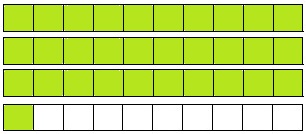
    — — 3 rectangles, each divided into 10 parts. 3 rectangles are fully shaded. 1 rectangle has 1 part shaded.
    [latex]\dfrac{9}{2}[/latex] — —
    — [latex]3\dfrac{1}{3}[/latex] —

     

  8. Convert each improper fraction to a mixed number or a whole number.
    1. [latex]\dfrac{9}{5}=[/latex]
    2. [latex]\dfrac{7}{2}=[/latex]
    3. [latex]\dfrac{11}{2}=[/latex]
    4. [latex]\dfrac{14}{5}=[/latex]
    5. [latex]\dfrac{4}{3}=[/latex]
    6. [latex]\dfrac{11}{6}=[/latex]
    7. [latex]\dfrac{7}{3}=[/latex]
    8. [latex]\dfrac{8}{4}=[/latex]

     

  9. Convert each whole number to an improper fraction. Use the denominator given to you.
    1. [latex]6=\dfrac{}{3}[/latex]
    2. [latex]5=\dfrac{}{2}[/latex]
    3. [latex]3=\dfrac{}{7}[/latex]
    4. [latex]7=\dfrac{}{3}[/latex]
    5. [latex]8=\dfrac{}{7}[/latex]
    6. [latex]1=\dfrac{}{3}[/latex]
    7. [latex]2=\dfrac{}{10}[/latex]

     

  10. Convert each mixed number to an improper fraction.
    1. [latex]6\dfrac{7}{8}=[/latex]
    2. [latex]2\dfrac{1}{2}=[/latex]
    3. [latex]15\dfrac{4}{5}=[/latex]
    4. [latex]20\dfrac{3}{7}=[/latex]
    5. [latex]18\dfrac{1}{2}=[/latex]
    6. [latex]5\dfrac{9}{11}=[/latex]

3.4: Review Answers

  1. Write fractions from the pictures.
    1. [latex]\dfrac{1}{4}[/latex]
    2. [latex]\dfrac{2}{3}[/latex]
    3. [latex]\dfrac{3}{4}[/latex]
    4. [latex]\dfrac{1}{2}[/latex]
    5. [latex]\dfrac{2}{4}[/latex]
    6. [latex]\dfrac{4}{6}[/latex]
    7. [latex]\dfrac{8}{10}[/latex]
  2. Draw your own fractions.
  3. Write the following fractions in words.
    1. one half
    2. one fourth or one quarter
    3. three fourths or three quarters
    4. twenty-one twenty fifths
    5. one third
    6. five sixths

     

  4. Answer the questions using a common fraction.
    1. [latex]\dfrac{20}{60}[/latex]
    2. [latex]\dfrac{5}{30}[/latex]
    3. [latex]\dfrac{6}{8}[/latex]
    4. [latex]\dfrac{6}{60}[/latex]
    5. [latex]\dfrac{300}{450}[/latex]
    6. [latex]\dfrac{30}{32}[/latex]

     

  5. Compare the following fractions:
    1. >
    2. >
    3. <
    4. <
    5. >
    6. <
    7. >
    8. <

     

  6. Identify each fraction by writing: proper fraction, improper fraction, or mixed number to each fraction.
    1. proper fraction
    2. improper fraction
    3. proper fraction
    4. improper fraction
    5. mixed number

     

  7. Fill in the missing parts of the chart (see Table 3.4.2).
    Table 3.4.2: Questions 7 Answers
    Improper
    Fraction    		 Mixed
    Number    		 Drawing
    [latex]\dfrac{11}{3}[/latex] [latex]3\dfrac{2}{3}[/latex] 4 rectangles each divided into 3 parts. 3 rectangles are fully shaded. One rectangle has 2 parts shaded.
    [latex]\dfrac{29}{6}[/latex] [latex]4\dfrac{5}{6}[/latex] 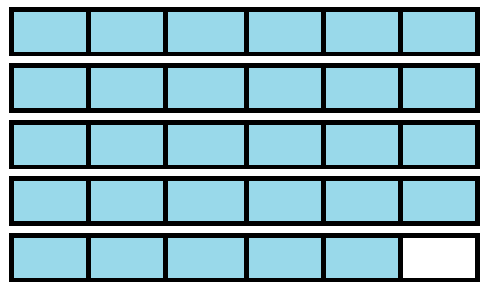5 rectangles divided into 6 parts. 4 rectangles are fully shaded. 1 rectangle has 5 parts shaded.
    [latex]\dfrac{11}{4}[/latex] [latex]2\dfrac{3}{4}[/latex] 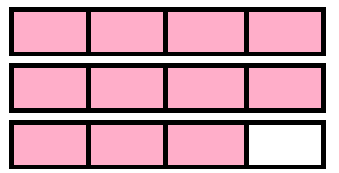3 rectangles each divided into 4 parts. 2 rectangles are fully shaded. 1 rectangle has 3 parts shaded.
    [latex]\dfrac{31}{10}[/latex] [latex]3\dfrac{1}{10}[/latex] 3 rectangles, each divided into 10 parts. 3 rectangles are fully shaded. 1 rectangle has 1 part shaded.
    [latex]\dfrac{9}{2}[/latex] [latex]4\dfrac{1}{2}[/latex] 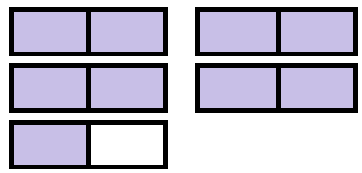5 rectangles divided in half. 4 rectangles are fully shaded. 1 rectangle is half shaded.
    [latex]\dfrac{10}{3}[/latex] [latex]3\dfrac{1}{3}[/latex] 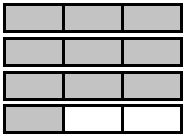4 rectangles each divided into 3 parts. 3 rectangles are fully shaded. One rectangle has 1 part shaded.

     

  8. Convert each improper fraction to a mixed number or a whole number.
    1. [latex]1\dfrac{4}{5}[/latex]
    2. [latex]3\dfrac{1}{2}[/latex]
    3. [latex]5\dfrac{1}{2}[/latex]
    4. [latex]2\dfrac{4}{5}[/latex]
    5. [latex]1\dfrac{1}{3}[/latex]
    6. [latex]1\dfrac{5}{6}[/latex]
    7. [latex]2\dfrac{1}{3}[/latex]
    8. [latex]2[/latex]

     

  9. Convert each whole number to an improper fraction. Use the denominator given to you.
    1. [latex]\dfrac{28}{3}[/latex]
    2. [latex]\dfrac{10}{2}[/latex]
    3. [latex]\dfrac{21}{7}[/latex]
    4. [latex]\dfrac{21}{3}[/latex]
    5. [latex]\dfrac{56}{7}[/latex]
    6. [latex]\dfrac{3}{3}[/latex]
    7. [latex]\dfrac{20}{10}[/latex]

     

  10. Convert each mixed number to an improper fraction.
    1. [latex]\dfrac{55}{8}[/latex]
    2. [latex]\dfrac{5}{2}[/latex]
    3. [latex]\dfrac{79}{5}[/latex]
    4. [latex]\dfrac{143}{7}[/latex]
    5. [latex]\dfrac{37}{2}[/latex]
    6. [latex]\dfrac{64}{11}[/latex]

Attribution

All figures in this chapter are from Unit 1 Review in Adult Literacy Fundamental Mathematics: Book 5 – 2nd Edition by Liz Girard, Wendy Tagami, and Leanne Caillier-Smith, via BCcampus.

This chapter has been adapted from Unit 1 Review in Adult Literacy Fundamental Mathematics: Book 5 – 2nd Edition (BCcampus) by Liz Girard, Wendy Tagami, and Leanne Caillier-Smith (2023), which is under a CC BY 4.0 license.

License

Icon for the Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License

Intermediate PreAlgebra: Building Success Copyright © 2024 by Kim Tamblyn, TRU Open Press is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License, except where otherwise noted.

Share This Book